Research
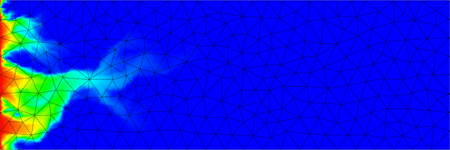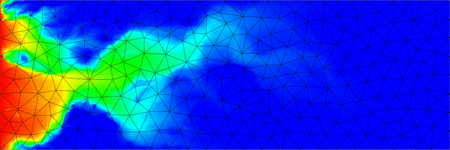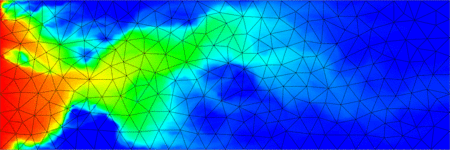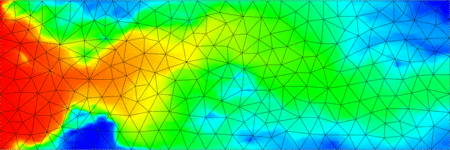
MHM METHODS
A new family of finite element methods, named Multiscale Hybrid-Mixed method (or MHM for short), aims to solve boundary value problems with multiscale coefficients on coarse meshes. The underlying upscaling procedure transfer to the basis functions the responsibility of achieving high orders of accuracy. The upscaling is built inside the general framework of hybridization, in which the continuity of the solution is relaxed a priori and imposed weakly through the action of Lagrange multipliers. We apply the MHM methodology to a hydrodynamic model (Darcy equation) and a transport model (reaction-advection-diffusion equation). This characterizes the unknowns as the solutions of local problems with Neumann and Robin boundary conditions driven by the multipliers, respectively. Such local problems are independent of one another, yielding a process naturally shaped for parallelization. Moreover, the multiscale decomposition indicates a new adaptivity algorithm to set up local spaces defined using a face-based a-posteriori estimator. Please visit the 'publications' section. New! MHM in the chilean newspapers